Fourier: The Mathematician of Heat

Joseph Fourier, often regarded as the Mathematician of Heat, made significant contributions to the fields of mathematics and physics, particularly in the study of heat conduction. His work on Fourier series and the heat equation revolutionized the understanding of heat transfer and laid the foundation for modern thermodynamics.

Early Life and Education

This article explores the life and contributions of Joseph Fourier, a prominent mathematician known for his work on heat conduction and the development of Fourier series, which revolutionized the field of mathematics and physics.

Joseph Fourier was born in 1768 in Auxerre, France, in a humble family. Despite facing financial struggles, he displayed exceptional talent in mathematics from a young age. Fourier's academic journey began at the local Benedictine abbey school, where his passion for numbers and patterns started to flourish.

After completing his primary education, Fourier continued his studies at the Collège Mazarin in Paris. It was here that his mathematical prowess caught the attention of renowned scholars, paving the way for his future endeavors in the realm of mathematics and science.

Driven by a thirst for knowledge, Fourier immersed himself in various subjects, ranging from geometry to physics. His insatiable curiosity and dedication to learning laid the foundation for his groundbreaking research on heat conduction and the formulation of the heat equation.

Despite facing numerous challenges along the way, including the tumultuous political landscape of post-Revolutionary France, Fourier's unwavering determination and intellectual acumen propelled him towards becoming one of the most influential mathematicians of his time.

Learn about Fourier's groundbreaking research on heat transfer and his formulation of the heat equation, a fundamental concept in thermodynamics.

Explore the significance of Fourier series in representing periodic functions and their applications in various branches of mathematics and engineering.

Understand Fourier's lasting impact on the field of mathematics, including his influence on the development of mathematical analysis and differential equations.

Delve into the concept of the Fourier transform and its utility in signal processing, image analysis, and other scientific disciplines.

Learn about Fourier's legacy in the scientific community and the numerous honors and accolades bestowed upon him for his contributions.

Examine Fourier's theorem and its implications for the representation of functions as infinite sums of sines and cosines.

Discover how Fourier's work continues to impact modern technologies and research across various fields, from telecommunications to medical imaging.

Stay tuned for answers to commonly asked questions about Joseph Fourier and his groundbreaking contributions to mathematics and science.

Research on Heat Conduction

Joseph Fourier's research on heat conduction marked a significant milestone in the field of thermodynamics. By studying the flow of heat through various materials, Fourier was able to develop a mathematical framework that laid the foundation for understanding thermal dynamics. His work on heat conduction led to the formulation of the heat equation, which remains a fundamental concept in physics and engineering.

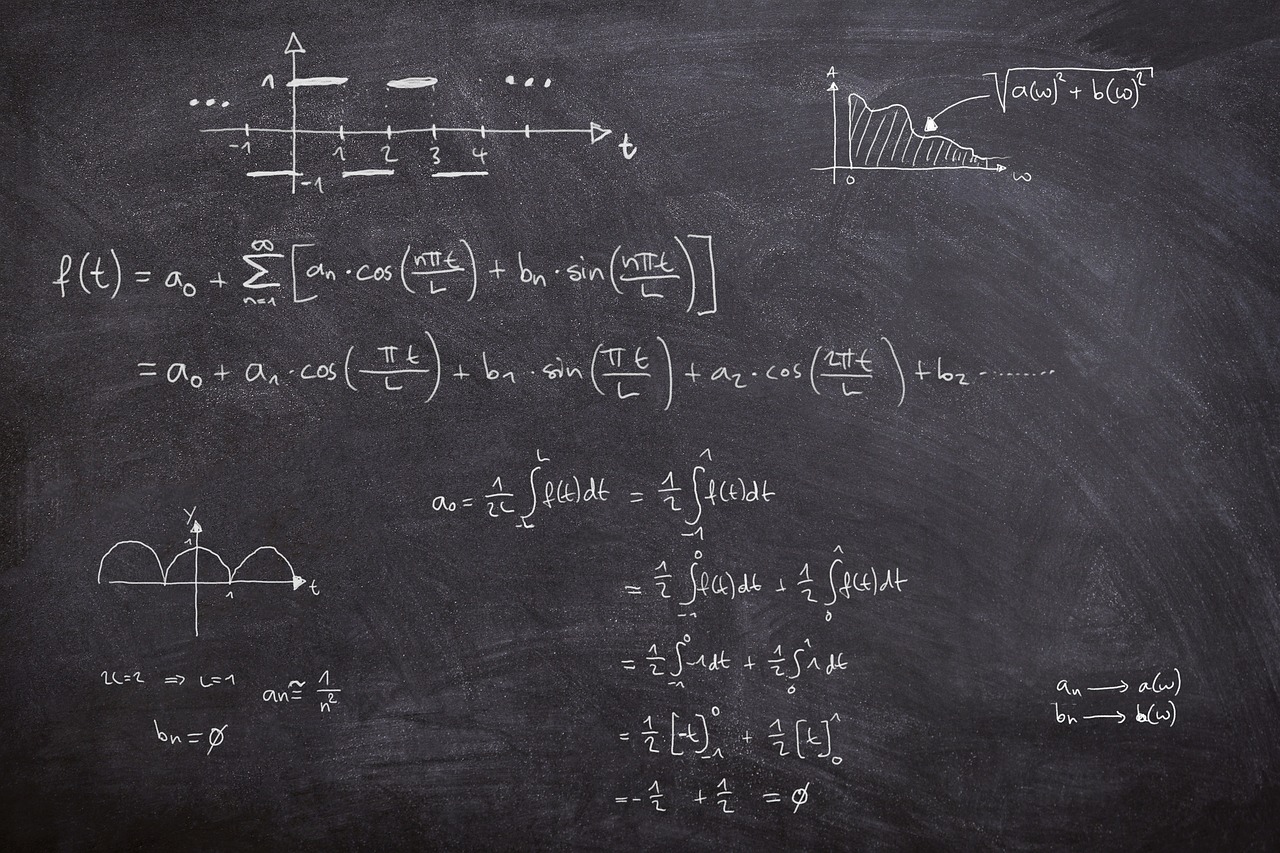
One of the key aspects of Fourier's research on heat conduction was his realization that complex temperature distributions could be represented as a sum of simple trigonometric functions. This insight eventually led to the development of Fourier series, a powerful tool for analyzing periodic phenomena in mathematics and physics.

Through his studies, Fourier demonstrated the interconnected nature of heat transfer and mathematical analysis. By applying his mathematical techniques to the study of heat conduction, he was able to uncover patterns and relationships that were previously unseen. This innovative approach not only advanced the field of thermodynamics but also paved the way for new developments in mathematical theory.

Furthermore, Fourier's research on heat conduction had practical implications beyond the realm of pure mathematics. His findings were instrumental in improving the efficiency of heat transfer processes in engineering applications, leading to advancements in areas such as thermal insulation and energy conservation.

Fourier Series

The is a powerful mathematical tool developed by Joseph Fourier that allows us to represent periodic functions as infinite sums of sine and cosine functions. This revolutionary concept has found wide applications in various fields, from engineering to physics, enabling the analysis and manipulation of complex waveforms with ease.

Imagine Fourier series as a versatile toolbox that can break down intricate patterns into simpler components, much like how a skilled chef deconstructs a complex recipe into individual ingredients. By decomposing a function into its fundamental building blocks, Fourier series provide a systematic approach to understanding and manipulating periodic phenomena.

One of the key strengths of Fourier series lies in their ability to approximate a wide range of functions through a combination of sine and cosine terms. This flexibility allows researchers and engineers to model diverse phenomena, from sound waves to electrical signals, with remarkable accuracy and efficiency.

Furthermore, the convergence properties of Fourier series offer valuable insights into the behavior of periodic functions, shedding light on their regularity and smoothness. This mathematical framework not only facilitates the analysis of complex systems but also provides a deeper understanding of the underlying dynamics governing natural processes.

Through the elegant formulation of Fourier series, Joseph Fourier laid the foundation for a new paradigm in mathematical analysis, transforming the way we perceive and manipulate periodic functions. As we continue to explore the applications of Fourier series in diverse fields, we honor the ingenuity and foresight of this pioneering mathematician whose legacy continues to inspire and enlighten generations of scholars and researchers.

Impact on Mathematics

Joseph Fourier's impact on mathematics is undeniable, as his work transcended the boundaries of traditional mathematical concepts and paved the way for new advancements in the field. By introducing the Fourier series, he revolutionized the representation of periodic functions, allowing for complex waveforms to be expressed as simple combinations of sines and cosines. This breakthrough not only simplified mathematical analysis but also found applications in diverse areas such as signal processing, engineering, and physics.

Moreover, Fourier's contributions extended to the realm of differential equations, where his research on heat conduction led to the development of the heat equation. This fundamental concept remains a cornerstone of thermodynamics and has influenced the study of various physical phenomena related to heat transfer. By formulating mathematical models to describe these processes, Fourier's work laid the groundwork for future generations of mathematicians and scientists to explore complex systems with greater precision and accuracy.

One of the most significant legacies of Fourier's impact on mathematics is the Fourier transform, a powerful mathematical tool that allows signals and functions to be analyzed in the frequency domain. This transformative technique has become indispensable in fields such as telecommunications, image processing, and data analysis, enabling researchers to extract valuable insights from complex datasets and signals. The versatility of the Fourier transform has made it a cornerstone of modern mathematics and a testament to Fourier's enduring influence on the field.

Fourier Transform

The is a powerful mathematical tool that allows us to analyze functions and signals in the frequency domain. By decomposing a function into its constituent frequencies, the Fourier Transform provides valuable insights into the behavior and characteristics of the original signal. This transformation is widely used in various scientific and engineering applications, such as signal processing, image analysis, and data compression.

When applying the Fourier Transform, a time-domain signal is converted into a frequency-domain representation, revealing the individual sinusoidal components that make up the signal. This process enables us to analyze the frequency content of a signal, identify dominant frequencies, and extract meaningful information for further processing or analysis.

One of the key advantages of the Fourier Transform is its ability to simplify complex mathematical operations by transforming them into the frequency domain, where certain calculations become more straightforward. This transformation facilitates tasks such as filtering, noise removal, and pattern recognition, making it an essential tool in modern technology and scientific research.

Legacy and Recognition

Joseph Fourier's legacy in the realm of mathematics and science is nothing short of monumental. His contributions have left an indelible mark on the scientific community, earning him widespread recognition and numerous accolades for his groundbreaking work. Fourier's innovative research on heat conduction and the development of the Fourier series have forever changed the landscape of mathematics and physics.

One of Fourier's most notable achievements is his formulation of the heat equation, a fundamental concept in thermodynamics that continues to be studied and applied in various scientific disciplines. This pivotal work laid the foundation for understanding heat transfer and paved the way for advancements in engineering and physics.

Furthermore, Fourier's exploration of periodic functions led to the development of the Fourier series, a powerful tool for representing complex functions as infinite sums of sines and cosines. This mathematical framework has found widespread applications in fields such as signal processing, image analysis, and telecommunications, showcasing the enduring relevance of Fourier's work in modern technologies.

Throughout his career, Fourier's dedication to advancing the frontiers of mathematics has earned him the respect and admiration of his peers. His theorem on representing functions as trigonometric series has become a cornerstone of mathematical analysis, demonstrating the depth of his contributions to the field.

Despite facing challenges and skepticism during his lifetime, Fourier's perseverance and intellectual prowess ultimately solidified his place as a pioneering figure in the history of mathematics. His legacy continues to inspire generations of mathematicians and scientists, underscoring the lasting impact of his innovative ideas and profound insights.

Fourier's Theorem

Fourier's Theorem, formulated by Joseph Fourier, is a fundamental concept in mathematics that revolutionized the representation of functions. It states that periodic functions can be expressed as an infinite sum of sines and cosines. This theorem provides a powerful tool for analyzing and understanding complex periodic phenomena, allowing for the decomposition of intricate functions into simpler components.

By applying Fourier's Theorem, mathematicians and scientists can break down intricate waveforms, signals, and patterns into their fundamental frequency components. This decomposition enables the study of various phenomena in fields such as signal processing, physics, and engineering, providing insights into the underlying structures and behaviors of complex systems.

Through Fourier's Theorem, the representation of functions as combinations of sine and cosine functions has found widespread applications in diverse areas, including telecommunications, audio processing, and image compression. The ability to analyze and manipulate signals using Fourier analysis has significantly advanced modern technology, leading to innovations in data transmission, digital media, and scientific research.

Overall, Fourier's Theorem stands as a cornerstone in mathematics and engineering, offering a powerful framework for understanding the intricate relationships between different components of complex systems and paving the way for groundbreaking discoveries and technological advancements.

Modern Applications

Modern Applications of Fourier's work are vast and diverse, permeating various aspects of our technological landscape. One prominent application is in telecommunications, where Fourier analysis plays a crucial role in signal processing, enabling the efficient transmission of data through different channels. The ability to decompose complex signals into simpler components using Fourier techniques has revolutionized communication systems, ensuring clear and reliable information exchange.

Moreover, Fourier transforms find extensive use in medical imaging, allowing healthcare professionals to obtain detailed images of internal structures non-invasively. Techniques such as MRI and CT scans heavily rely on Fourier analysis to reconstruct images from raw data, providing valuable insights for diagnosis and treatment planning. The ability to extract meaningful information from complex signals has significantly advanced the field of medical imaging, enhancing patient care and outcomes.

Furthermore, Fourier's principles are integral to audio processing technologies, shaping the way we record, manipulate, and reproduce sound. From music production to speech recognition systems, Fourier analysis enables the decomposition of audio signals into frequency components, facilitating tasks such as noise reduction, equalization, and compression. The widespread adoption of Fourier techniques in audio engineering has elevated the quality and efficiency of audio-related applications, enriching our auditory experiences.

Frequently Asked Questions

• What are Fourier series?

  Fourier series are sets of trigonometric functions that can represent periodic functions as an infinite sum of sines and cosines. They have wide applications in various fields such as signal processing, engineering, and physics.

• How did Joseph Fourier contribute to mathematics?

  Joseph Fourier made significant contributions to mathematics by introducing the concept of Fourier series, which revolutionized the representation of periodic functions. He also developed the Fourier transform, a powerful tool used in signal processing and scientific research.

• What is the significance of Fourier's research on heat conduction?

  Fourier's research on heat conduction led to the formulation of the heat equation, a fundamental concept in thermodynamics. His work laid the foundation for understanding the transfer of heat in various materials and systems.

• What are some modern applications of Fourier's work?

  Fourier's work continues to have a profound impact on modern technologies, including telecommunications, medical imaging, and data compression. His theories are widely used in fields that require the analysis and processing of signals and images.